基于canvas使用贝塞尔曲线平滑拟合折线段

[Aaaaaaaty]

写在最前

本次分享一下在canvas中将绘制出来的折线段的棱角“磨平”，也就是通过贝塞尔曲线穿过各个描点来代替原有的折线图。

欢迎关注我的博客，不定期更新中——

为什么要平滑拟合折线段

先来看下Echarts下折线图的渲染效果：

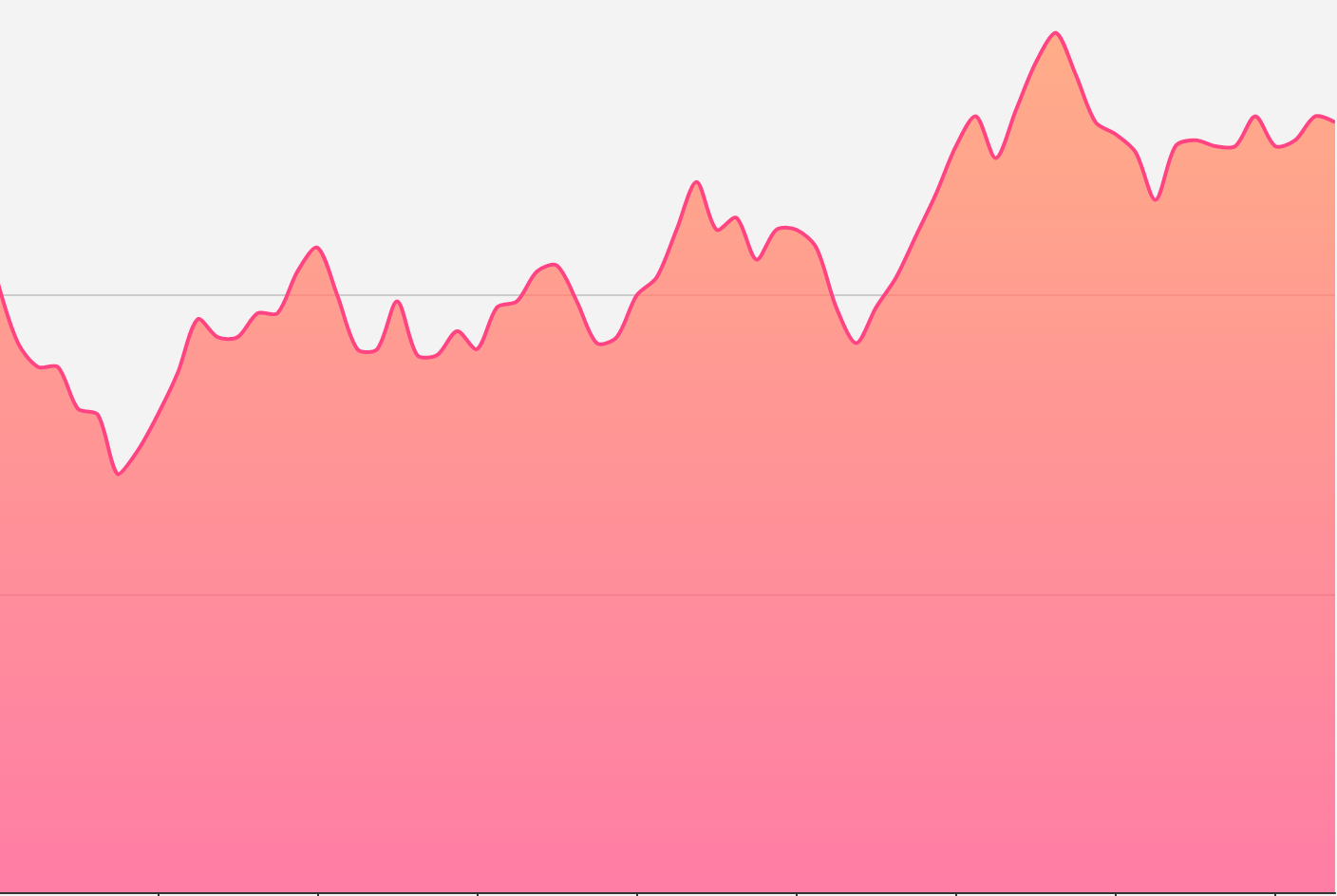
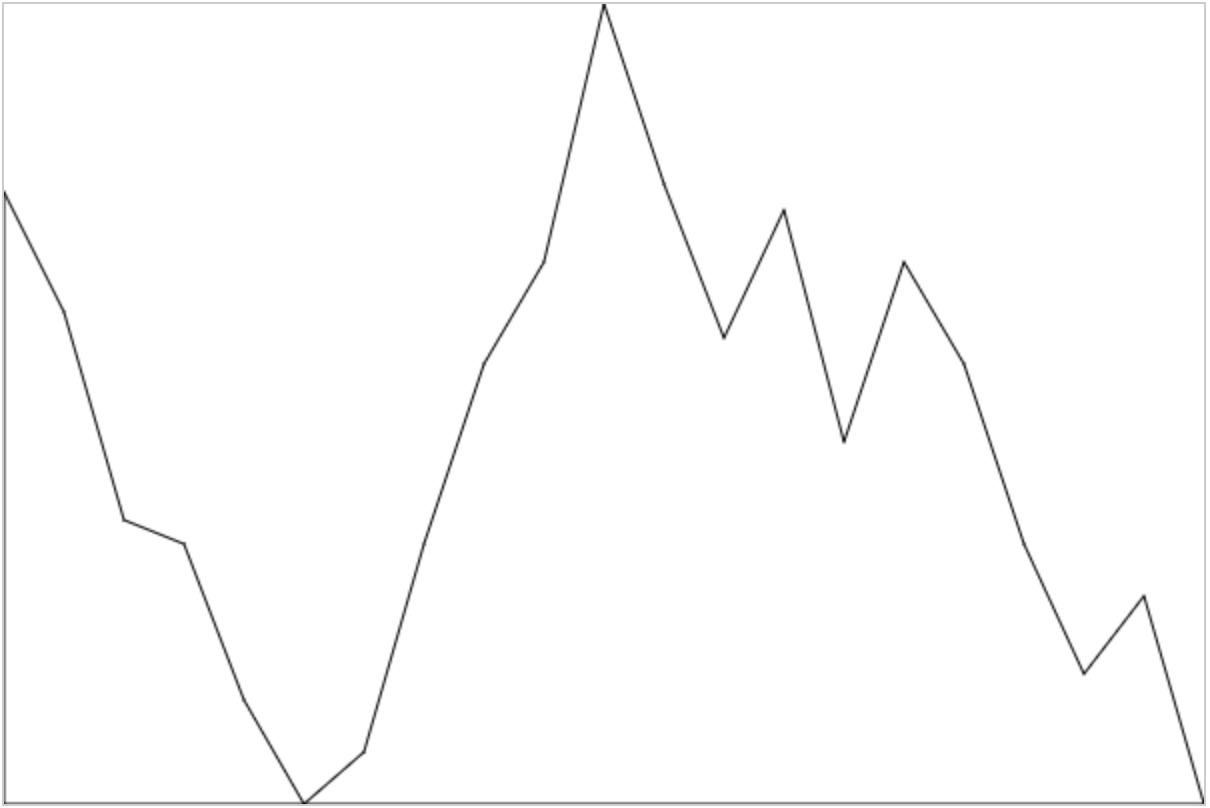
一开始我没注意到其实这个折线段是曲线穿过去的，只认为是单纯的描点绘图，所以起初我实现的“简(丑)易(陋)”版本是这样的：

不要关注样式，重点就是实现之后才发现看起来人家Echarts的实现描点非常的圆滑，也由此引发了之后的探讨。怎么有规律的画平滑曲线？

效果图

先来看下最终模仿的实现：
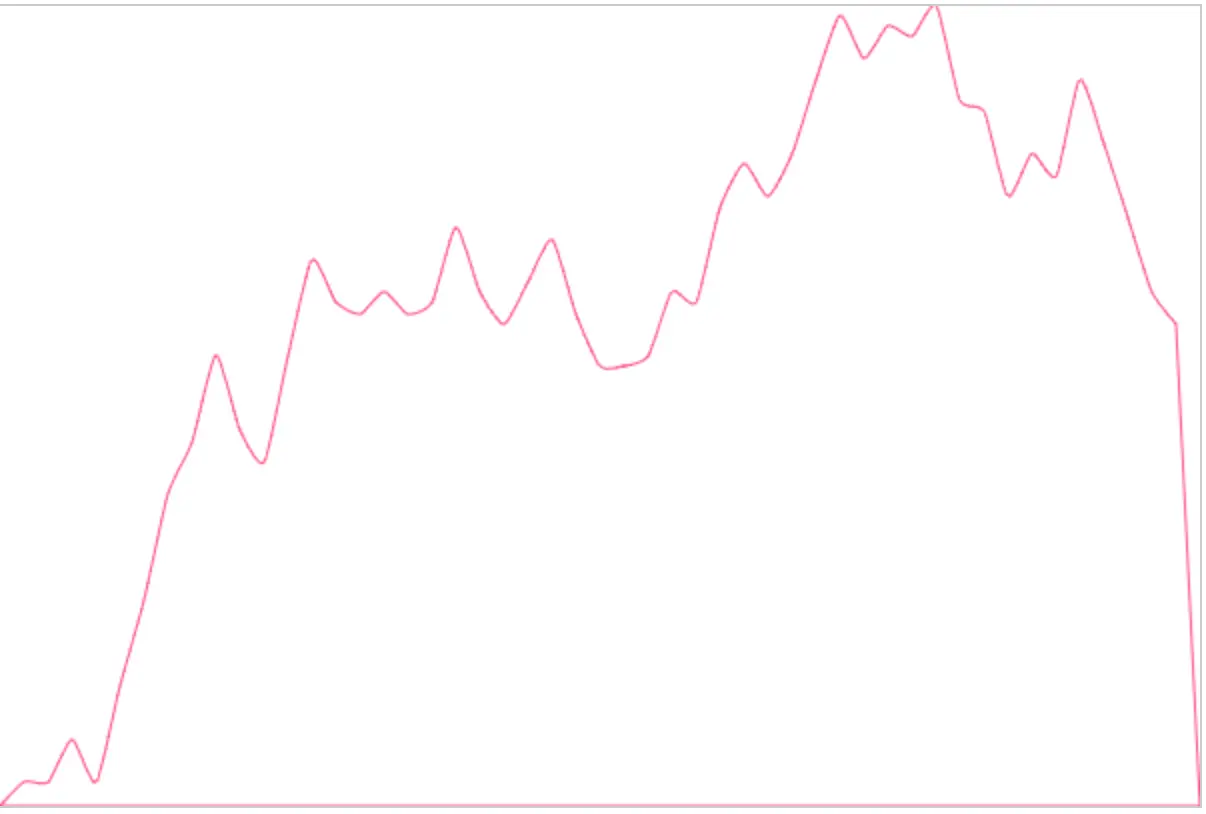
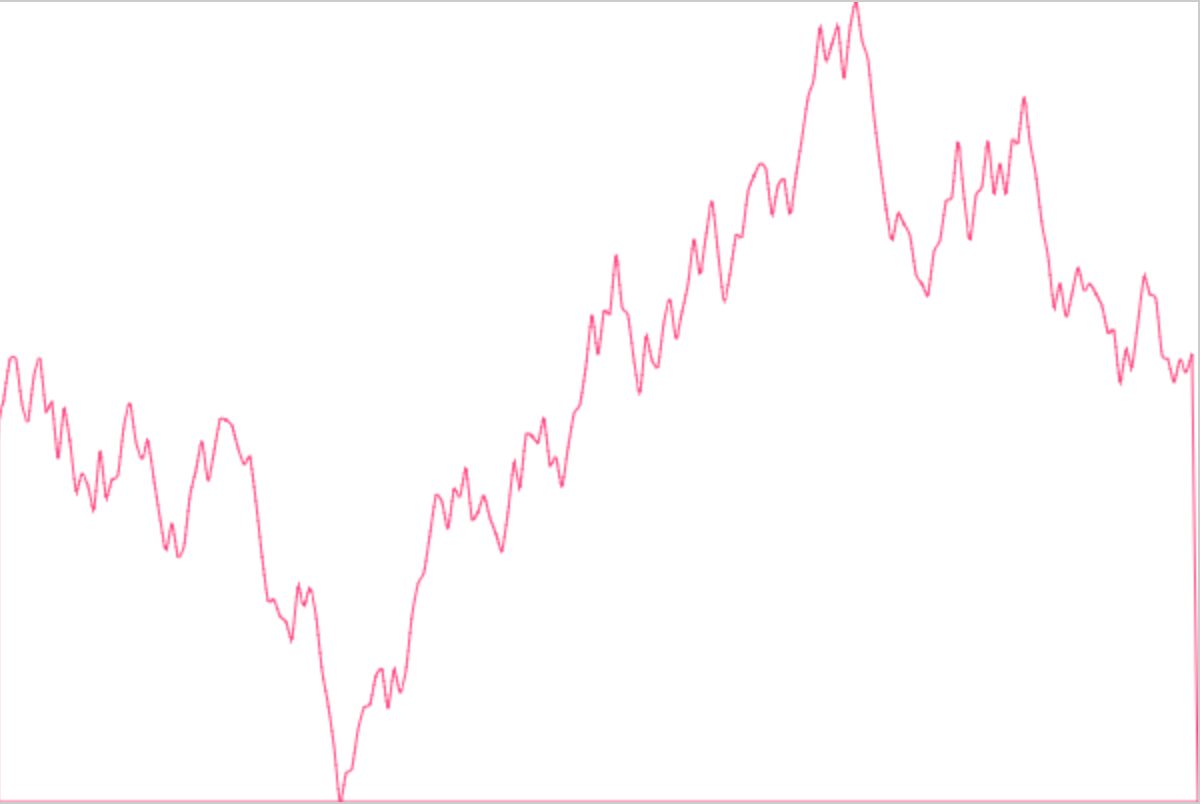
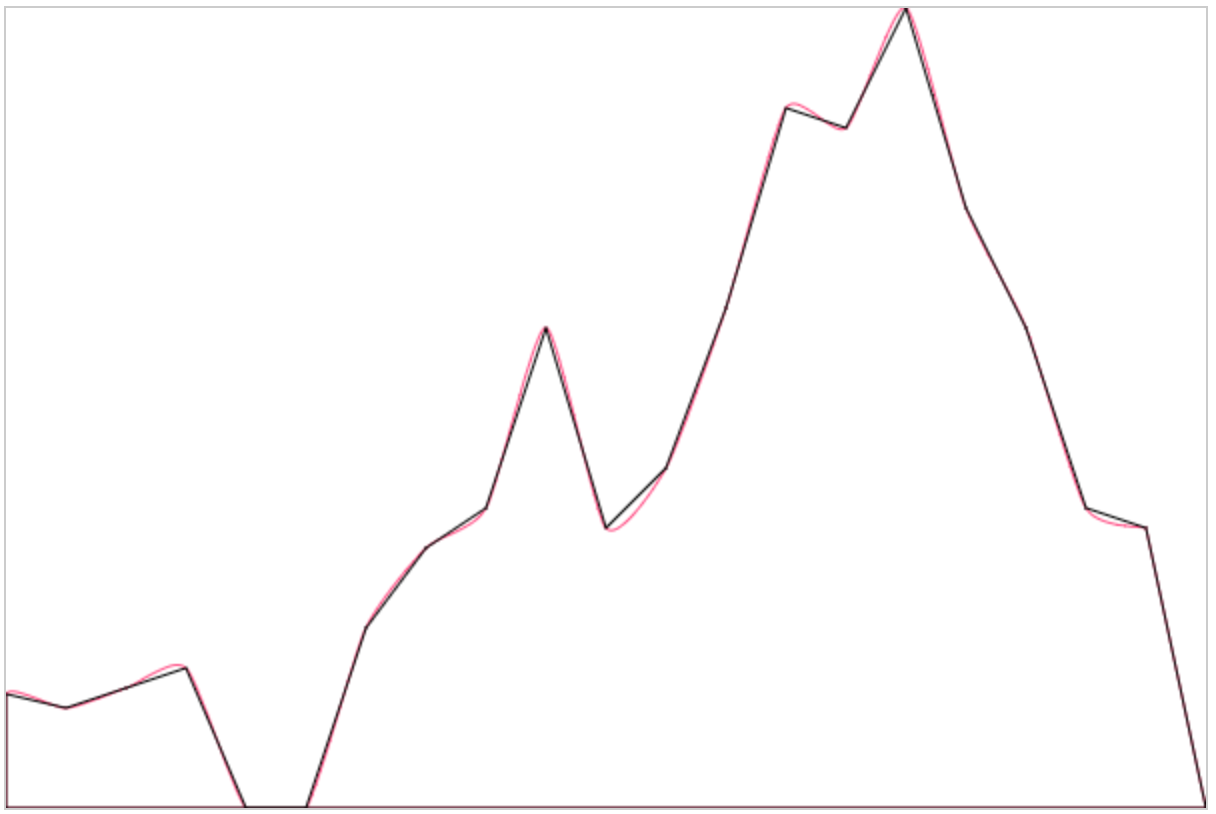
因为我也不知道Echarts内部怎么实现的（逃


看起来已经非常圆润了，和我们最初的设想十分接近了。再看下曲线是否穿过了描点：

好的！结果很明显现在来重新看下我们的实现方式。

实现过程

• 绘制折线图
• 贝塞尔曲线平滑拟合

模拟数据

var data = [Math.random() * 300];
        for (var i = 1; i < 50; i++) { //按照echarts
            data.push(Math.round((Math.random() - 0.5) * 20 + data[i - 1]));
        }
        option = {
            canvas:{
                id: 'canvas'
            },
            series: {
                name: '模拟数据',
                itemStyle: {
                    color: 'rgb(255, 70, 131)'
                },
                areaStyle: {
                    color: 'rgb(255, 158, 68)'
                },
                data: data
            }
        };

绘制折线图

首先初始化一个构造函数来放置需要用到的数据：

 function LinearGradient(option) {
    this.canvas = document.getElementById(option.canvas.id)
    this.ctx = this.canvas.getContext('2d')
    this.width = this.canvas.width
    this.height = this.canvas.height
    this.tooltip = option.tooltip
    this.title = option.text
    this.series = option.series //存放模拟数据
}

绘制折线图：

LinearGradient.prototype.draw1 = function() { //折线参考线
    ... 
    //要考虑到canvas中的原点是左上角，
    //所以下面要做一些换算，
    //diff为x,y轴被数据最大值和最小值的取值范围所平分的等份。
    this.series.data.forEach(function(item, index) {
        var x = diffX * index,
            y = Math.floor(self.height - diffY * (item - dataMin))
        self.ctx.lineTo(x, y) //绘制各个数据点
    })
    ...
}

贝塞尔曲线平滑拟合

贝塞尔曲线的关键点在于控制点的选择，这个网站可以动态的展现控制点不同而绘制的不同的曲线。而对于控制点的计算。。作者还是选择了百度一下毕竟数学不好：），这篇文章对于将多个点使用贝塞尔曲线连接时各个控制点的计算。具体算法有兴趣的同学可以深入了解下，现在直接说下计算控制点的结论。

上面的公式涉及到四个坐标点，当前点，前一个点以及后两个点，而当坐标值为下图展示的时候绘制出来的曲线如下所示：

不过会有一个问题就是起始点和最后一个点不能用这个公式，不过那篇文章也给出了边界值的处理办法：

所以在将折线换成平滑曲线的时候，将边界值以及其他控制点计算好之后代入到贝塞尔函数中就完成了：

//核心实现
this.series.data.forEach(function(item, index) { //找到前一个点到下一个点中间的控制点
    var scale = 0.1 //分别对于ab控制点的一个正数，可以分别自行调整
    var last1X = diffX * (index - 1),
        last1Y = Math.floor(self.height - diffY * (self.series.data[index - 1] - dataMin)),
        //前一个点坐标
        last2X = diffX * (index - 2),
        last2Y = Math.floor(self.height - diffY * (self.series.data[index - 2] - dataMin)),
        //前两个点坐标
        nowX = diffX * (index),
        nowY = Math.floor(self.height - diffY * (self.series.data[index] - dataMin)),
        //当期点坐标
        nextX = diffX * (index + 1),
        nextY = Math.floor(self.height - diffY * (self.series.data[index + 1] - dataMin)),
        //下一个点坐标
        cAx = last1X + (nowX - last2X) * scale,
        cAy = last1Y + (nowY - last2Y) * scale,
        cBx = nowX - (nextX - last1X) * scale,
        cBy = nowY - (nextY - last1Y) * scale 
    if(index === 0) {
        self.ctx.lineTo(nowX, nowY)
        return
    } else if(index ===1) {
        cAx = last1X + (nowX - 0) * scale
        cAy = last1Y + (nowY - self.height) * scale 
    } else if(index === self.series.data.length - 1) {
        cBx = nowX - (nowX - last1X) * scale
        cBy = nowY - (nowY - last1Y) * scale
    } 
        self.ctx.bezierCurveTo(cAx, cAy, cBx, cBy, nowX, nowY);
        //绘制出上一个点到当前点的贝塞尔曲线
    })

由于我每次遍历的点都是当前点，但是文章中给出的公式是计算会知道下一个点的控制点算法，故在代码实现中我将所有点的计算挪前了一位。当index = 0时也就是初始点是不需要曲线绘制的，因为我们绘制的是从前一个点到当前点的曲线，没有到0的曲线需要绘制。从index = 1开始我们就可以正常开始绘制，从0到1的曲线，由于index = 1时是没有在他前面第二个点的故其属于边界值点，也就是需要特殊进行计算，以及最后一个点。其余均按照正常公式算出AB的xy坐标代入贝塞尔函数即可。

参考文章

• 平滑曲线生成：贝塞尔曲线拟合
• 贝塞尔曲线控制点确定的方法

最后

源代码见这里
惯例po作者的博客，不定时更新中——
有问题欢迎在issues下交流。

[GreendaMi]

1. PathEffect mEffect = new CornerPathEffect(20);//平滑过渡的角度
   2.mPaint.setPathEffect(mEffect);
   3.canvas.drawPath(path, mPaint);
   直接用path实现，不用计算

[Aaaaaaaty]

@GreendaMi 大佬我百度了一下这个写法好像是安卓的0.0 我不太会233 我是用的html5的canvas..

[GreendaMi]

@Aaaaaaaty 打扰了，看起来像Android的kotlin

[Aaaaaaaty]

@GreendaMi 受教了受教了

[jellenm]

持续关注中....

[TigerCouple]

hi，请问，你的github的blog是用什么写的啊？markdown么？

[Aaaaaaaty]

@TigerCouple markdown

[wangfpp]

diff是啥意思 没看明白

[Paxosgold]

• Good morning team great job keep it up ****